What is the difference between Scalar field and Vector Field?

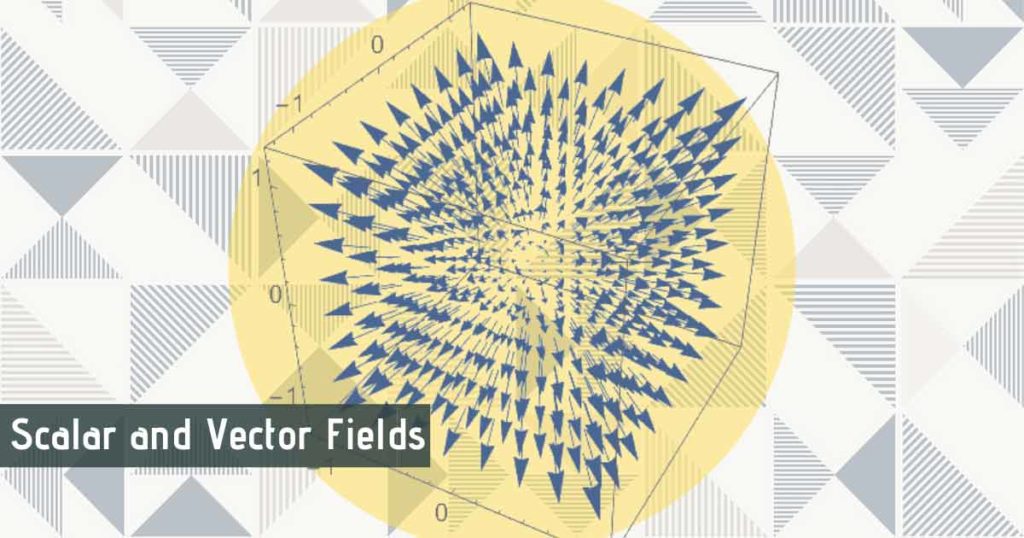
Scalar field and Vector field are basic concepts whose proper understanding is necessary for the study of Electromagnetics. This article explains their definitions and difference.

What do we mean by field?

In general, the word “Field” is synonym for “Function” in Mathematics. So field is a composite function of different variables.

What is the Scalar Field?

In the 3D space, each point can be represented by proper coordinate system. For Cartesian coordinate system it would be (x, y, z).

So the function, f(x, y, z) is called as the Scalar field. For example, V=x^2+yz. Here V can be called as the Scalar field.

Consider a cube or 3D space as shown in the following figure.

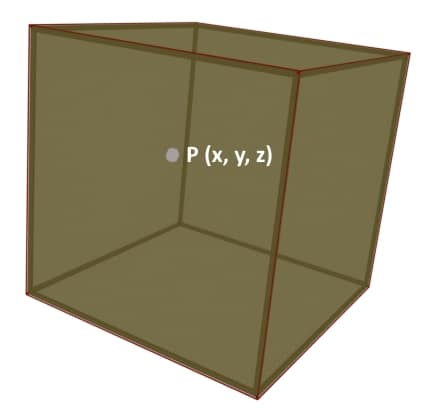
Every point of this cube can be represented as (x, y, z). Let us say the inside temperature is given as, T=x^2+yz. This temperature, T is the scalar field.

The temperature of each point inside the cube can be found out by putting x, y and z values in the function. For example, at (1, 2, 3) the value is given as, T=1^2+(2)(3)=7. And likewise for every point inside. So the value of the field, in our case the temperature, is different for the different points. If we use a colour code, like dark colour for the higher value of the field and light one for lower field value; then we can certain gradient pattern. This can be the representation of the Scalar field. The following figure is the actual simulation of certain Scalar function.

Potential, Work, Energy etc are the examples of the Scalar fields. The Gradient operation is performed on the Scalar fields.

What is the Vector Field?

Consider the same cube above where each point inside is represented as (x, y, z). Let us say we are given with the function as follows: –

\overrightarrow A=f(x,y,z){\overrightarrow a}_x+f(x,y,z){\overrightarrow a}_y+f(x,y,z){\overrightarrow a}_z

Such function, having components of the vector as a function or combination of constants and function, is known as the Vector field.

Again consider that we are given with the Electric field inside the cube as, \overrightarrow E=x^2{\overrightarrow a}_x+{4yz{\overrightarrow a}_y}-10{xy{\overrightarrow a}_z}. This is a vector field.

The Electric field at every point inside the cube can be found out by putting x, y and z values. For example, E at (1, 2, 3) is

\overrightarrow E=1^2{\overrightarrow a}_x+{4(2)(3){\overrightarrow a}_y}-10{(1)(2){\overrightarrow a}_z={\overrightarrow a}_x+24{\overrightarrow a}_y-20{\overrightarrow a}_z}

So at each point within the space, there is a vector having a certain magnitude and the direction. The pictorial representation of certain vector field using actual simulation is as follows: –

An Electric field, Magnetic field, Force field etc. are the few examples of the vector field. The Divergence and Curl operations are performed on such fields.