Ohmic Power in the expression of Poynting Theorem, explained in a simple manner.

Poynting Theorem is one of the key topics that we study under EM wave propagation. It gives us the EM wave power. While deriving the Poynting Theorem, we come across the term volume integration of sigme into E square. This we call as an Ohmic Power dissipated in the given volume. Let’s see, why?

Power of EM wave and Poynting Theorem

The Poynting theorem, named after the physicist John Henry Poynting, is a fundamental principle in electromagnetism that describes the flow of energy in an electromagnetic field.

Poynting theorem states that the total power flowing through a given surface is equal to the rate of decrease of the electromagnetic energy density within that volume plus the ohmic power loss in that volume.

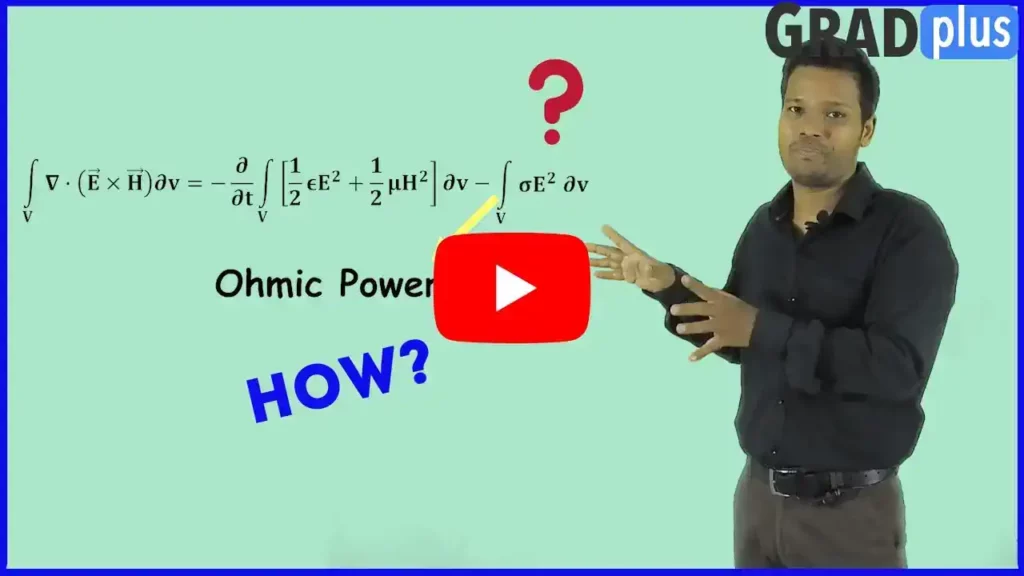
-\oint_s\left(\overrightarrow E\times\overrightarrow H\right)\cdot\overrightarrow{ds}=\frac\partial{\partial t}\int_v\left[\frac12\varepsilon E^2+\frac12\mu H^2\right]dv+\int_v\sigma E^2dv

The first term on the right hand side is total electromagnetic energy increased in the given volume.

But, our point of concern is second term. We call it as ohmic power dissipated within the given volume. Let’s see why?

\int_v\sigma E^2dv

This term is an ohmic power. And this can be proved by multiple ways. But let’s select simple one.

We will try to find the unit for this term. It must come as Watt to call that term as power. Isn’t it?

σ is the conductivity while E is an electric field.

We know that σE is nothing but the conduction current density i.e. J.

So, we can write this term \int_v\sigma E^2dv as \int_v\overrightarrow J\cdot\overrightarrow Edv.

Now the unit for J is A/m².

While, the unit for E is V/m.

Hence, unit for \overrightarrow J\cdot\overrightarrow E is \frac{A\times V}{m^3}.

Now we have volume integrationof this term. So, m³ will get cancelled.

So, the unit for \int_v\sigma E^2dv is Amp-Volt i.e. Watt.

So, this term must represent power. And that power must be called as an ohmic power. Why?

Because as we study in the networks, the power dissipated due to resistivity or conducvity is nothing but the ohmic nature.

Hence, the term \int_v\sigma E^2dv is an ohmic power. Simple!